ROCKET STABILITY
by Vernon Estes NAR #380
One of the first principles any rocket designer must learn is that a rocket will fly only if the center of gravity is ahead of the center of pressure far enough to allow the air currents to cause a stabilizing effect.
From your science class or other scientific studies,
you probably have learned that if a rotating force is
applied to a free body in space it will cause it to
rotate around its center of gravity. As an example of
this, you could take a wooden dowel or uniform stick
about two feet long and toss it into the air in a
manner in which the
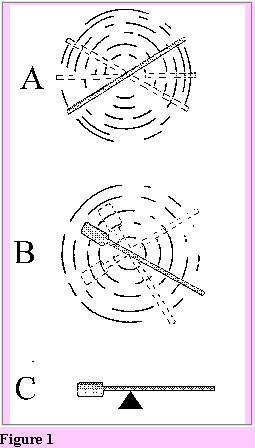stick will have an end over end
rotating type motion. (See Figure 1, Example A) You
will notice that regardless of how you throw the stick,
vertically or horizontally, hard or easy, it will always
rotate about its center. If a weight is then attached to
one end of the stick and it is again thrown into the air
it will rotate about a new location. (Figure 1,
Example B) This time the point about which it rotates
will be closer to the weighted end. If you take the
weighted stick and balance it across a sharp edge you
will find that the point about which it balances (its
center of gravity) is the same point about which it had
been rotating when tossed into the air. (Figure 1,
Example C)
This simple explanation should aid you in understanding how a free body in space rotates around its center of gravity. A model rocket in flight is a free body in "space". If, for any reason, a force is applied to the flying rocket to cause it to rotate, it will always do so about its center of gravity.
Rotating forces applied to rockets in flight can result from lateral winds, air drag on nose cones, weights off center, air drag on launch lugs, crooked fins, effect of exhaust gasses emitted at an angle, motor mounted at an angle of slightly off center, unbalanced drag on fins, unequal streamlining, etc. Obviously, some of these factors are going to be present in all rockets. Therefore, since rotating forces will be present, your rocket must be designed to overcome them. If your rocket is not so designed it will loop around and go "everywhere" but end up going nowhere. Nearly all model rockets are stabilized by air currents. By stabilized, we mean that all rotating forces are counteracted or overcome. This means that for each force trying to make the rocket rotate we must set up an equal and opposite force to counteract it.
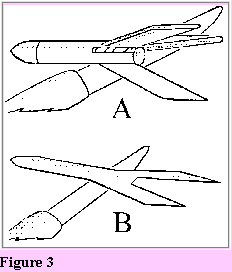
How is this accomplished? Ask any rocket expert and he will simply say to design the rocket so that the center of gravity is ahead of the center of pressure. From studying our first experiment it is easy to see how we could find the center of gravity by simply balancing the rocket on a knife edge as shown in Example A of Figure 3. But what and where is the center of pressure? The following experiment should aid you in understanding more about the center of pressure of a rocket.
Suppose we take the same 2 foot long piece of dowel
used in our first experiment and place it on a low friction
pivot as shown in Example A of Figure 2. Then suppose
the dowel is held in a uniform air current (wind) of 10 or
15 miles per hour. If the pivot has been placed in the
center of the dowel and if the dowel is uniform in size
(area) the forces exerted by the air pressure will be equal
on both sides of the pivot and the air current will
produce no rotating effect. If, however, a vane of some
sort, such as a 3" x 3" piece of cardboard, is glued to one
end of the dowel and it is again put into the air stream
with the
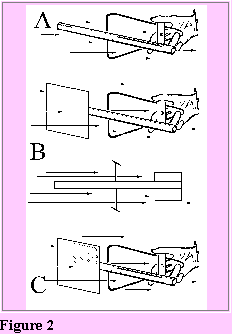pivot in the same position, the moving air
current will apply the greatest force against the end of the
dowel which has the vane attached to it. (See Example B,
Figure 2) This will cause the dowel to rotate so that the
end away from the vane points into the wind. If we now
move the pivot closer to the vane end of the dowel we
will be able to locate a point along the dowel where equal
air pressure will be applied to both ends. The air current
will no longer cause rotation. This point is called the
lateral center of pressure. Remember , the center of pressure has to do only with the forces
applied by air currents, and the larger the surface the greater the forces will be.
The ideal way to find the lateral center of pressure of a
model rocket is to suspend the rocket between pivots as
was done with the 2 foot dowel in Figure 2,
and subject
the rocket to a uniform lateral air current. This can be
accomplished to some degree of accuracy by holding the
suspended rocket in a breeze of 10 to 15 M.P.H., or, on a
still day, running with the suspended rocket. The same
effect can be accomplished very accurately by the use of a
low velocity wind tunnel. However, since most of you
model rocket builders and designers will not have wind
tunnels and mechanisms for suspending your rocket on a
low friction pivot, another method must be provided for
determining the center of pressure. Keeping in mind the
fact that the air pressure applied to a surface is
proportional to the area of the surface, it then becomes
possible to approximate the rotating effect of the action
of the air pressure by making a uniform area cutout of your rocket and locating the balancing
point of this cutout. To make this area cutout, simply lay your rocket over a piece of cardboard
and mark around the edges. Next, cut around the lines and balance the cutout on a knife edge as
shown in Example B of Figure 3.
The discussion just presented tells how to find the lateral center of pressure -the center of pressure with the air currents hitting the rocket broadside. As a rocket is rotated so the nose faces into the wind the forces produced by the air currents will also be affected by the thickness of the fins, shape of the nose cone, etc. This will cause the center of pressure of the rocket to shift, slightly, to a new location. However, with most designs this shift is of such effect as to add to the stability and therefore can safely be disregarded.
Suppose a model rocket starts to rotate in flight. It will rotate around its center of gravity. When it turns, the air rushing past it will then hit the rocket at an angle. If the center of pressure is behind the center of gravity on the model, the air pressure will exert the greatest force against the fins. This will counteract the rotating forces and the model will continue to fly straight. If, on the other hand, the center of pressure is ahead of the center of gravity the air current will exert a greater force against the nose end of the rocket. This will cause it to rotate ever farther, once it has begun rotating, and the model will go head over heels in the air.
What degree of stability is required? Experience has shown that if the center of gravity and the center of pressure are determined as outlined above, your rocket will have ample stability if the center of gravity is ahead of the center of pressure by at least one half the diameter (caliber) of your rocket.
Suppose you construct a rocket and find the above conditions have not been met. Do not try to fly it. Corrections must be made. It will not be stable and it could be dangerous. Tests have been made where the stability of the model rocket was in question. If it was completely unstable it would loop around and around in the air, seldom reaching over 30 feet in height and never reaching a velocity in excess of 20 or 30 miles per hour. However, occasionally one of these rockets would make a couple of loops, suddenly become stable due to the lessening of the fuel load, and make a bee line straight into the ground. Had anyone been standing in the wrong place a serious injury could have resulted.
If a rocket does not show the degree of stability required for safety it can be easily altered to conform either by moving the center of gravity forward or by moving the center of pressure rearward. To move the center of gravity forward, a heavier nose cone is used or a weight is added to the nose of the rocket. To move the center of pressure rearward, the fins can be made larger or moved farther back on the body tube. With many designs, greater stability is obtained by constructing it so that a large portion of the fins project beyond the rear of the rocket body.